August 12, 2023 report
This article has been reviewed according to Science X's editorial process and policies. Editors have highlighted the following attributes while ensuring the content's credibility:
fact-checked
peer-reviewed publication
trusted source
proofread
An algorithm that shapes objects to cause them to roll down ramps following a desired path
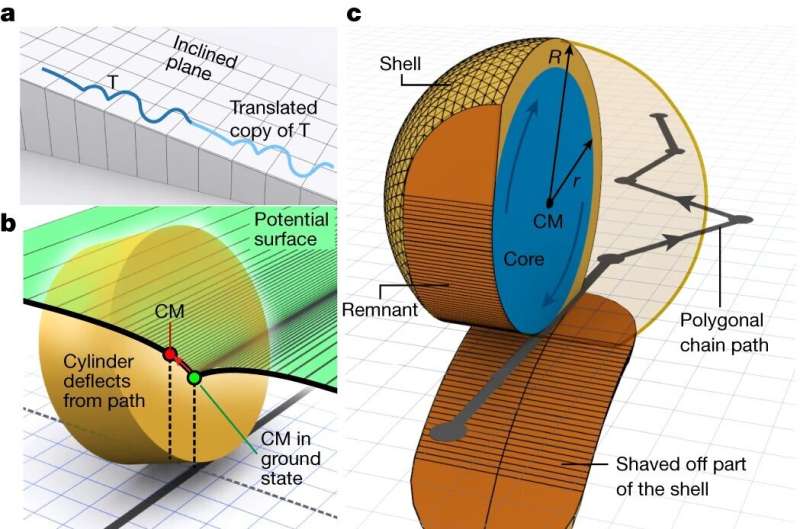
A team of physicists and mathematicians at the Institute for Basic Science's Center for Soft and Living Matter, in South Korea, working with a colleague from the University of Geneva, has developed an algorithm that can be used to find the shape of an object to cause it to roll down a ramp following a desired path.
In their paper published in the journal Nature, the group describes how they developed their algorithm, and possible uses for it. Elisabetta Matsumoto and Henry Segerman with the Georgia Institute of Technology and Oklahoma State University, respectively, have published a News & Views piece in the same journal issue outlining the work done by the team on this new effort.
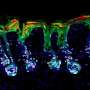
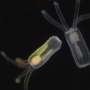
In this new effort, the research team started with an interesting puzzle—one that begins by envisioning a sphere rolling down a ramp. If the sphere is imagined to be made of clay, it can be manipulated (deformed) as it rolls to make it conform to a given path.
If the sphere is then rolled down the ramp again, it will follow the previous path due to the new deformities in its shape. The researchers noted that the paths that could be taken by the sphere could be nearly limitless due to the nearly limitless possible deformations.
That realization led them to wonder if the deformations that form in such a sphere could be corelated mathematically with its path. And if so, if such math could be used to create an algorithm that could be used to 3D print a sphere with deformations that would force it to follow a predetermined path.
It turned out the answer to both questions was yes. The team used math and physics principles to create formulas that described deformations to a given object that would result in the object following a desired path down an inclined plane. They then created a computer program that could be used to create such an object in the real world, using 3D printing.
The team named the objects trajectoids. Each had a solid metal ball-bearing inside to give it weight. They also found that they could create trajectoids that traveled over a given path twice, and named them "two-period trajectoids."
The research team suggests their formulas and algorithm could be used in robotics applications and also in physics research associated with the angular moment of an electron—or in quantum research centered around the study of evolution of a quantum bit.
More information: Yaroslav I. Sobolev et al, Solid-body trajectoids shaped to roll along desired pathways, Nature (2023). DOI: 10.1038/s41586-023-06306-y
Elisabetta Matsumoto et al, Shaped to roll along a programmed periodic path, Nature (2023). DOI: 10.1038/d41586-023-02335-9
Journal information: Nature
© 2023 Science X Network